I started my unit on Geometry with my 6th graders before Christmas break.  We got as far as the basic vocabulary and different types of angles.  I decided to begin with polygons after break, so my first post-Christmas lesson was on triangles.

We started off by discussing the different ways to classify triangles – by their sides and by their angles.  The students made a chart in their notebook listing the different types of triangles and we did some example problems.

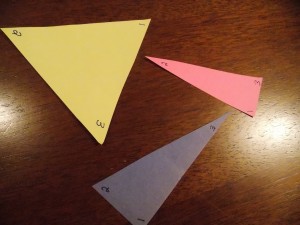
[Before the lesson, I had made up little slips of paper with different triangle types on them.]  After going over the basics, I had each student pick one of the slips at random.

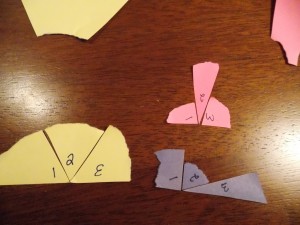
They had to draw whatever kind of triangle they picked on construction paper and then cut it out.  This served two purposes – it showed me if they understood the first part of the lesson, and it provided me with a wide variety of triangles.  I had each student number the angles in their triangle (1, 2, and 3).  I then asked the students to rip off each of the angles of their triangle.  Finally, I asked the students to line up their three angles so that they were adjacent to each other, and asked them what they noticed when they arranged their angles in that way.  A few students noted that they formed a straight line.

When I asked the students how many degrees the three angles must be in all if they are forming a straight line, the light bulbs went off!  Since everyone had started with a different triangle, the students were able to conclude that the angle sum of ANY triangle is 180 degrees.

All in all the lesson went very well.  We finished by going through an example of finding the missing angle in a triangle.  The students’ notes for the day ended up looking like this:

At the beginning of the class when we were first going over types of triangles, one of the students had asked why an acute triangle has 3 acute angles, but a right triangle only has 1 right angle and an obtuse triangle only has 1 obtuse angle.  I posed the question back to the class and their original answer was that the triangle wouldn’t close if it had 2 right angles or 2 obtuse angles.  At the end of class, however, one of the boys in my class said “Oh, that’s why there can only be 1 right or obtuse angle…there’s only 180 degrees in all so if you already have 90 degrees, if you had another 90 degree angle you wouldn’t be able to fit another angle!”

Don’t you just love it when students can reason out the answers to their own questions?!

Thanks for reading,

Christina