Today’s 6th grade lesson was on rotational symmetry.  I have found that students tend to be less familiar and less comfortable with rotational symmetry than they are with line symmetry (and sometimes mix the two up), so I try to find ways to make it more clear and understandable to them.

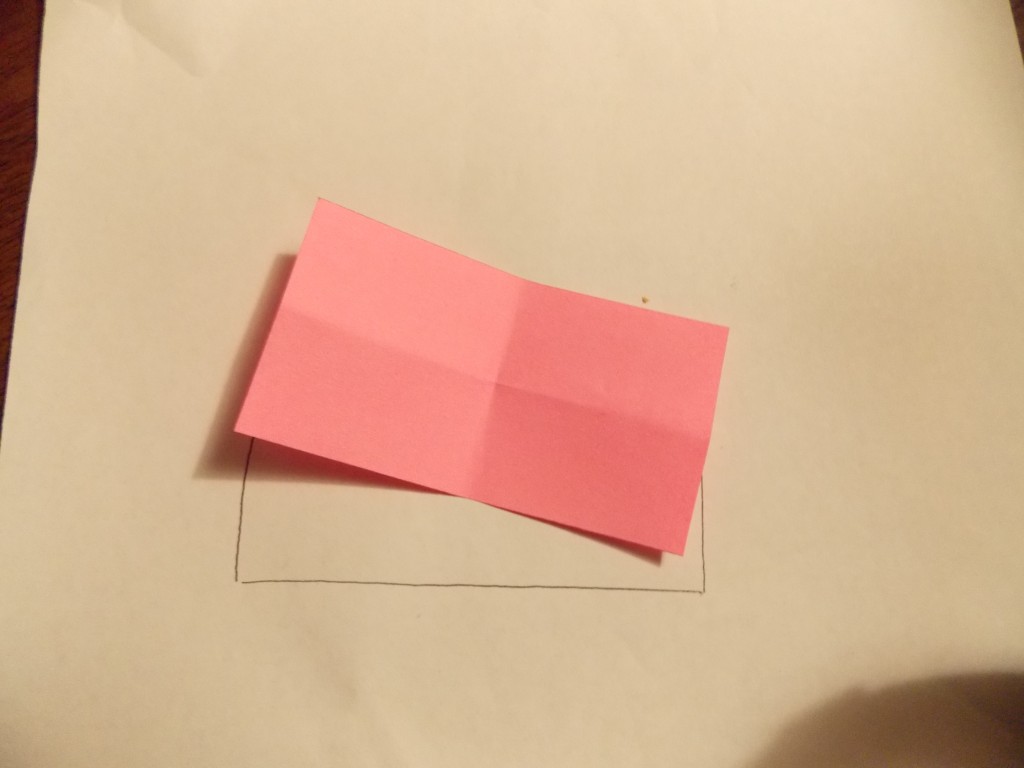
I had several different shapes cut out ahead of time: an equilateral triangle, isosceles triangle, rectangle, square, rhombus, regular pentagon, irregular hexagon, etc.  I had the students each pick a couple of different shapes and trace them on a piece of paper.

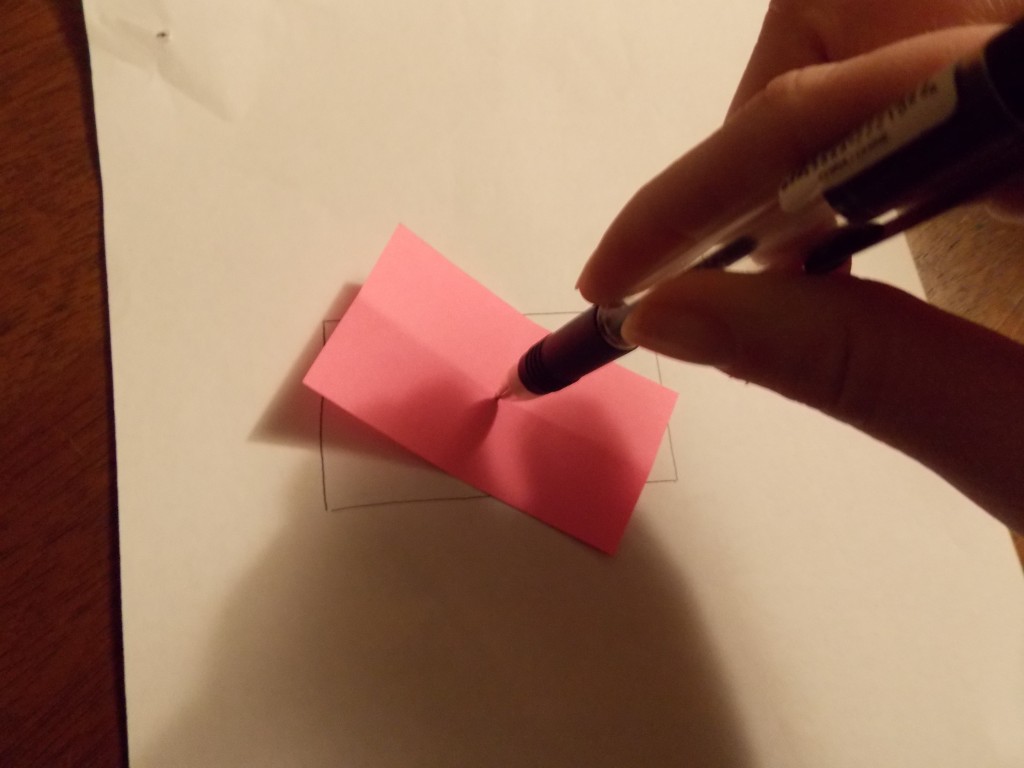
After tracing the shapes, the students were told to use their pen or pencil to hold down the center of the shape.  I had them rotate the shapes and count how many times the cut out shape lined up perfectly with the traced shape (until they got to a full turn around).

I asked the students to try to figure out how many degrees they were able to turn the figure to have it line up with their tracing.  They were able to reason that since a full turn was 360 degrees, they had to divide the number of times they could turn the shape into 360.

Their finished notes for the day looked like this:

As an extension, I gave each of the students an angle measure.  They have to draw, color, and cut out their own figure that has that rotational symmetry.  (For example, the student to which I assigned 120 degrees is not allowed to draw an equilateral triangle.  They have to create their own, original figure that also has 120 degree rotational symmetry).

I am excited to see what they come up with!

Thanks for reading,

Christina