Multiplying and dividing integers are, in my opinion, the hardest operations to teach to students and it took me a few years before I found a good way to have these operations make sense to students.  After I am confident that my students are comfortable adding and subtracting integers, I move on to the final two operations.  (Click here for my post on teaching integer addition.  Click here for my post on integer subtraction.)

I begin with a class discussion of what multiplication means.  Once the students are able to tell me that multiplying means “grouping,” I pull out the two-color counters again…

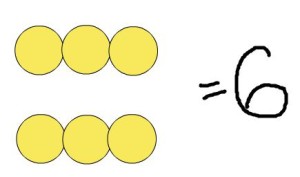
I start with a positive x positive:  2 x 3.  We discuss how this means “make 2 groups of 3”.  The students show me this with their counters and get 6 as an answer.

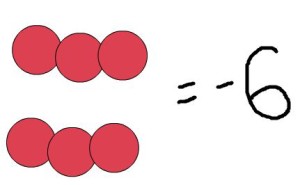
I move onto a positive x negative problem: 2 x (-3).  This means “make 2 groups of -3”. The students are able to do this easily with the two-color counters, as well, by making 2 groups of 3 red counters to get an answer of -6.

Now we get to harder questions:  negative x positive and negative x negative.  I ask the class “if a positive times a number means to make groups, what do you think a negative times a number means?”  Then we come up with the idea that a negative times a number must mean to take away groups.

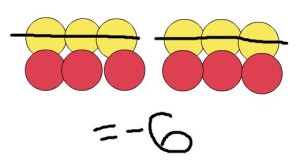
So we do a negative x positive problem: -2 x 3, which means “take away 2 groups of 3”.  Obviously the students can’t take away groups when there are no groups to begin with, so I ask the students what to do.  Because they are familiar with having to add zero pairs to take away numbers from our lesson on subtraction, the students are able to tell me that I will need to first make 6 zero pairs and then take away 2 groups of 3 yellow counters, leaving them with 6 red counters, or -6.

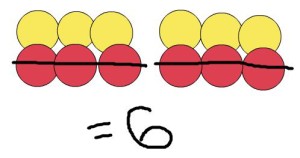
Finally, we move onto a negative x negative problem: -2 x (-3), meaning “take away 2 groups of -3”.  The students are able to figure this one out on their own based on the last example, so they again add 6 zero pairs, but this time take away 2 groups of 3 red counters, leaving them with 6 yellow counters (positive 6) as their answer.

I give the students problems to try on their own and then we come up with our formal rules for our notebooks:

Same signs:  multiply the absolute values of the numbers and make answer positive

Different signs:  multiply the absolute values of the numbers and make answer negative

Coming up with “real-world” examples for adding and subtracting integers is easy – you can use elevators moving up and down, temperatures rising and falling, and gains and losses in football.  It was much harder for me to come up with a “real-world” example for multiplication to give my students, but I finally have one that I am happy with that makes sense to the students and that I can use to show all 4 types of multiplication problems!

• Positive x Positive: It is 0 degrees outside.  The temperature is rising 2 degrees every hour.  What will the temperature be in 5 hours?  [The students know to do 2 x 5 = 10 degrees, so they are multiplying the rate the temperature is changing, which in this case is positive since the temperature is rising, by the time, which is also positive since I am talking about the future]
• Negative x Positive: It is 0 degrees outside.  The temperature is dropping 2 degrees every hour.  What will the temperature be in 5 hours?  [-2 x 5 = -10 degrees – the rate is negative since the temperature is dropping, and the time is positive]
•  Positive x Negative: It is 0 degrees outside.  The temperature rises 2 degrees every hour.  What was the temperature 5 hours ago?   [2 x (-5) = -10 degrees – the rate is positive, since the temperature is rising, but the time is negative since I am talking about going backwards in time]

• Negative x Negative: It is 0 degrees outside.  The temperature drops 2 degrees every hour.  What was the temperature 5 hours ago?  [-2 x (-5) = 10 degrees – both the rate and the time are negative]

 

I teach dividing integers just by using the inverse of multiplication.  For example, I give the problem -24 ÷ 4.   Since they already know multiplication of integers rules they simply ask themselves 4 x ? = -24, so the answer must be -6 since a positive x negative = negative.

Students then come to the conclusion that multiplication and division use the same rules since they are inverses.

Once the students understand the rules, they have no problem solving multiplication and division problems, as they find multiplying & dividing integers to be easier than adding and subtracting.  I have them complete worksheets and/or play review games with them for practice and then I move on to practicing all 4 operations together the next day.

Click the image below to download a FREE page students can add to their notebooks  for a quick reference on integer operations.

If you are looking for more resources on integer operations, check out my Integer Bundle, which includes 21 (differentiated) worksheets, 4 games, and 3 sets of task cards.

I would love to hear how other teachers teach integer operations, especially real-world examples for multiplication and/or division.  Please leave a comment if you would like to share!

 

Thanks for reading,

Christina