Whenever possible I like to have students discover or figure out the lesson on their own (with some guidance from me, of course), rather than simply teaching them an algorithm.  One such topic is factoring trinomials.

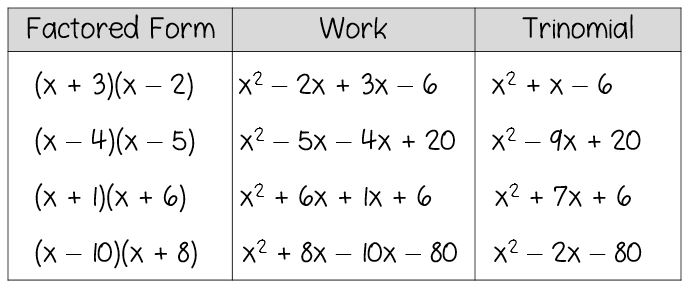
I start my lesson on factoring trinomials with a = 1 by giving students 4 binomial multiplication problems and having them solve them, showing all of their work.

I then tell them that factoring is the opposite of multiplying, so basically they are given the “answer” to a multiplication problem and they need to figure out the “problem”.  I have them look back at the previous 4 trinomial “answers” they got and to try to come up with a rule for factoring by figuring out where the b and c came from.

With a little time the students are always able to come up with the idea that the b is the sum of the 2^nd terms in the binomials and c is their product.  So, when I give them a trinomial to factor they know that they will have (x +/- #)(x +/-#) and need to find 2 numbers whose product is c and sum is b to fill in the #s.

I like teaching factoring this way because the students understand that I didn’t just make up some rule.  They came up with the rule themselves by analyzing problems that they already knew how to solve.

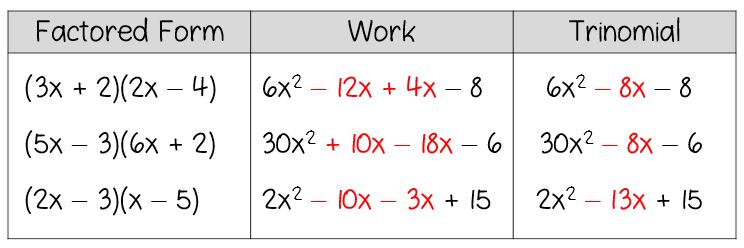
I also teach factoring trinomials where a ≠ 1 this way.

With these problems I have students come up with a rule to go from the trinomial answer to the “work” column.  They are usually pretty quick to notice that the first and last terms stay the same and that the middle term gets split into 2 terms.  When I ask how to know how to split up those middle terms they are able to come up with the idea that it needs to be split into 2 numbers whose sum is the original number but I usually have to push them to get them to see that the those 2 numbers also must have a product of ac.  I teach factoring by grouping earlier in the unit, so once students get to this point in the problem, they are able to just apply the factoring by grouping method to finish the problems.  (I know that there are other methods to factor trinomials where a ≠ 1, like guess & check and the “airplane” or “slip and slide” method but I personally find that factoring by grouping makes the most sense for students since they are using 2 things they already know: multiplication of binomials and the distributive property, in reverse.  I think this lends itself perfectly to helping students understand that factoring and multiplication are simply inverses).

Factoring is such an important part of algebra and is used in many different ways (solving quadratic equations, simplifying rational expressions, solving radical equations, etc.) that it is really important that students master it.  At least in my opinion, having students take some ownership of the process helps build their understanding and mastery.

[For students who understand the process of factoring but struggle to come up with the 2 numbers with the given sum and product I show them how to use a factor tree to help them find the numbers.  You can read about that here.]

Thanks for reading,

Christina